7.3.1 Inductive Sensing

An alternating current (AC) flowing through a coil generates an AC magnetic field. If a conductive material, such as a metal target, is brought into the vicinity of the coil, this magnetic field induces circulating currents (eddy currents) on the surface of the target. These eddy currents are a function of the distance, size, and composition of the target. These eddy currents then generate a magnetic field that opposes the original field generated by the coil. This mechanism is best compared to a transformer, where the coil is the primary core and the eddy current is the secondary core. The inductive coupling between both cores depends on distance and shape. Hence the resistance and inductance of the secondary core (eddy current), shows up as a distant dependent resistive and inductive component on the primary side (coil). Figure 5 through Figure 8 show a simplified circuit model.

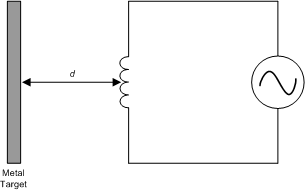Figure 5. Inductor With a Metal Target

Eddy currents generated on the surface of the target can be modeled as a transformer as shown in Figure 6. The coupling between the primary and secondary coils is a function of the distance and characteristics of the conductor. In Figure 6, the inductance L_s is the inductance of the coil, and r_s is the parasitic series resistance of the coil. The inductance L(d), which is a function of distance, d, is the coupled inductance of the metal target. Likewise, R(d) is the parasitic resistance of the eddy currents.

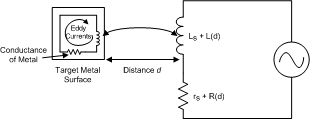Figure 6. Metal Target Modeled as L and R With Circulating Eddy Currents

Generating an alternating magnetic field with just an inductor consumes a large amount of power. This power consumption can be reduced by adding a parallel capacitor, turning the right part of Figure 6 into a resonator as shown in Figure 7. In this manner the power consumption is reduced to the eddy and inductor losses r_s + R(d) only.

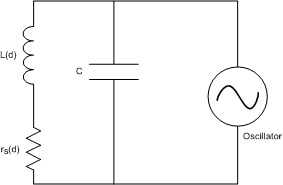Figure 7. LC Tank Connected to Oscillator

The LDC1001-Q1 device does not directly measure the series resistance. Instead, the device measures the equivalent parallel resonance impedance R_P (see Figure 8). This representation is equivalent to the representation shown in Figure 8, where the parallel resonance impedance R_P(d) is given by Equation 1.

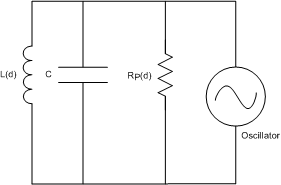Figure 8. Equivalent Resistance of r_s in Parallel With LC Tank

Figure 9 shows the variation in R_P as a function of distance for a 14-mm diameter PCB coil (23 turns, 4-mil trace width, 4-mil spacing between trace, 1-oz copper thickness, FR4). The target metal used is a stainless steel 2-mm thick.

Figure 9. Typical R_P vs Distance With a 14-mm PCB Coil