Although the OPA3S328 integrated switches offer relatively low parasitic capacitance and low on resistance, the designer needs to verify the effect of the switches to ensure the stability of the circuit. Using the same open-loop simulation circuit of Figure 4-2, we add the simplified switch model and plot the loop gain, AOL, and 1/Beta to analyze stability.

Figure 5-2 show the TINA-TI circuit schematic used to analyze stability incorporating the simplified switch model for each gain. Perform an AC transfer characteristic simulation and use the post-processor to generate the open-loop gain (AOL) and noise gain (1/β) curves for each gain setting. Figure 5-2 shows the stability analysis simulation results for R_F1 = 200kΩ, C_F1=1.6pF including the integrated switch capacitance.

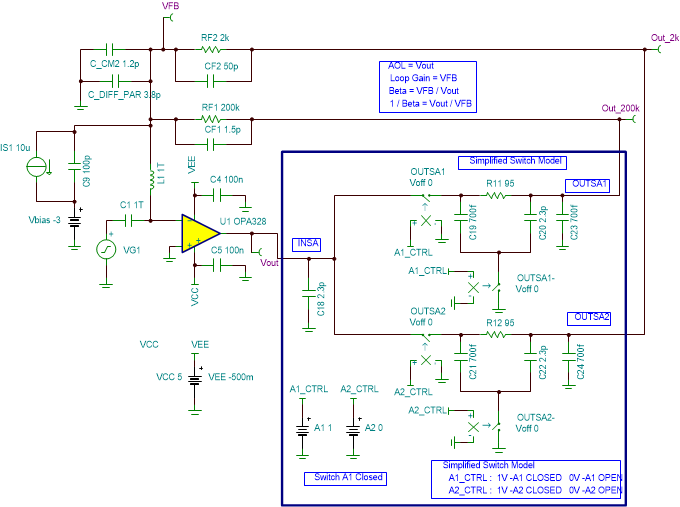Figure 5-2 TINA-TI Schematic for Stability Analysis Including Switches

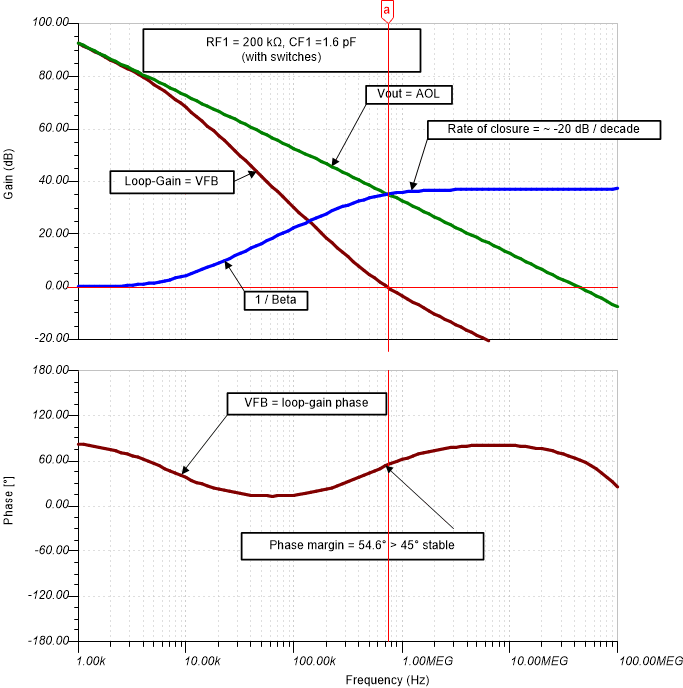Figure 5-3 Stability Analysis for R_F1 = 200kΩ, C_F1 = 1.6pF with Switch

After adding the switch parasitic components, the stability analysis for R_F1 = 200kΩ and C_F1 = 1.6pF shows the AOL and 1/β curves intersect with a rate of closure of -20 dB/decade. The phase margin remains higher than 45-degrees, guaranteeing robust circuit stability for this gain.

Figure 5-4 displays the results for R_F2= 2kΩ and C_F2=150pF.

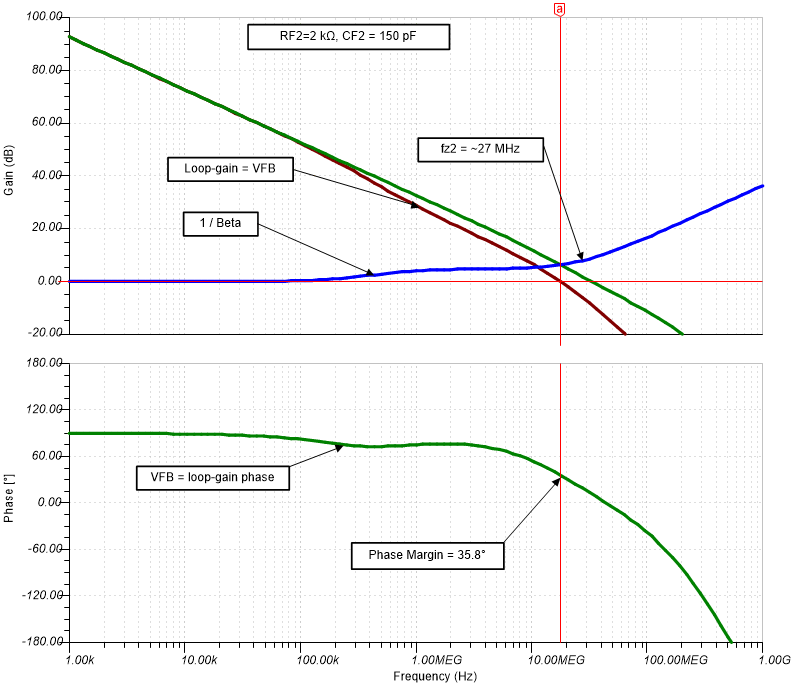Figure 5-4 Stability Analysis for R_F2 = 2kΩ, C_F2 = 150pF with Switch

The R_ON switch resistance interacts with the series combination of the feedback capacitor C_F2 and the total capacitance at the input of the amplifier C_T, generating an additional zero f_Z2 on the 1/β curve. Equation 11 defines the second zero frequency on the 1/β curve:

Equation 11.

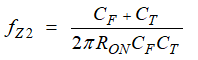
f_Z2 degrades the phase margin to ~36-degrees. The previous calculation in Table 4-1 and Table 4-2 shows that the permissible feedback capacitor range for C_F2 is between 16pF and 159pF. To improve phase margin, reduce the feedback capacitor C_F2 to 50pF, moving the second zero f_Z2, to a higher frequency than the amplifier's unity gain-bandwidth of 40-MHz, where the effect f_Z2 on the circuit stability is negligible. Figure 5-5 displays the stability analysis results for the revised circuit with R_F2= 2kΩ and C_F2=50pF. The phase margin improves to 57-degrees, guaranteeing robust circuit stability.

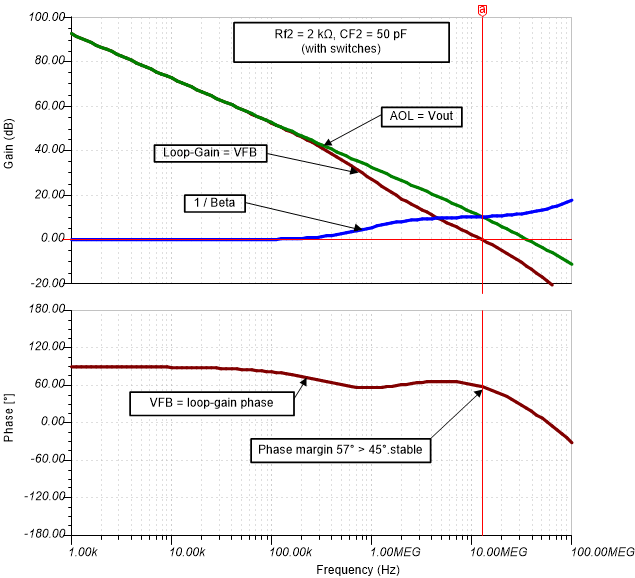Figure 5-5 Stability Analysis for Revised Circuit with R_F2 = 2kΩ, C_F2 = 50pF